Aim:How do we find the area of regular polygons?
March 19, 2012
The area of a regular polygon:
You can divide a regular polygon into congruent isosceles triangles by drawing segments to each vertex.
Base: S (side) Altitude: A (apothem)
The variable A appears in the formula for regular polygons. It stands for the length called the apothem, the perpendicular segment from the center to the side of the polygon.
Regular polygon area conjecture:
The area of a regular polygon is given by the formula 1/2nas, where A is the area, a is the apothem, n is the number of sides ans S is the length.
formula: 1/2nas or p= 1/2Pa
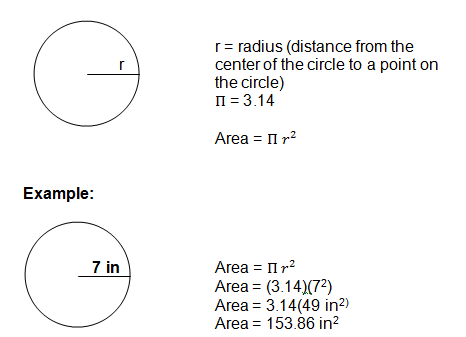
 The locus of points at a fixed distance, d, from point P is a circle with the given point P as its center and d as its radius.
The locus of points at a fixed distance, d, from point P is a circle with the given point P as its center and d as its radius.